Unit 4 (Fractions & Decimals)

Q.1 A number representing a part of a _________ is called a fraction.

Ans.
A number representing a part of a whole is called a fraction.
Example: ¼, ¾, 1/5, 3/6 etc.

Q.2 A fraction with denominator greater than the numerator is called a _________ fraction.

Ans.
A fraction with denominator greater than the numerator is called a proper fraction.
Example: 2/5, 3/8, 10/11 etc. are proper fractions.

Q.3 Fractions with the same denominator are called _________ fractions.

Ans.
Fractions with the same denominator are called like fractions.
Example: ½, 3/2, 5/2, 7/2 etc.

Q.4 18/5 is an fraction.

Ans.
18/5 is an improper fraction.
A fraction whose numerator is greater than the denominator is called an improper fraction.

Q.5 7/19 is a fraction.

Ans.
7/19 is a proper fraction.
A fraction whose numerator is less than the denominator is called a proper fraction.

Q.6 5/8 and 3/8 are proper fraction.

Ans.
5/8 and 3/8 are like proper fraction.
Fractions with same denominators are called like fractions.

Q.7 6/11 and 6/13 are proper fractions.

Ans.
6/11 and 6/13 are unlike proper fractions.
If the denominators are different, then they are called unlike fractions.

Q.8 The fraction 6/15 in simplest form is .

Ans.
The fraction 6/15 in simplest form is 2/5.
The given fraction 6/15, is further simplified by dividing both numerator and denominator by 3.
= 2/5

Q.9 The fraction 17/34 in simplest form is .

Ans.
The fraction 17/34 in simplest form is ½.
The given fraction 17/34, is further simplified by dividing both numerator and denominator by 17.
= ½

Q.10 18/135 and 90/675 are proper, unlike and fractions.

Ans.
18/135 and 90/675 are proper, unlike and equivalent fractions.
Consider the two given fractions, 18/135 and 90/675
So, (18/135) = (90/675)
By cross multiplication, we get
(18 × 675) = (90 × 135)
12,150 = 12,150
Therefore, 18/135 and 90/675 are proper, unlike and equivalent fractions.

Q.11  is equal to the improper fraction .

Ans.
is equal to the improper fraction 58/7.
Given mixed fraction is converted into improper fraction as = ((7 × 8) + 2)/7
= (56 + 2)/7
= 58/7

Q.12 87/7 is equal to the mixed fraction ______.

Ans.
87/7 is equal to the mixed fraction .
We know that, mixed fraction = Quotient Remainder/Divisor

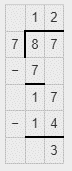
Therefore, 87/7 is equal to the mixed fraction .

Q.13 9 + (2/10) + (6/100) is equal to the decimal number ______.

Ans.
9 + (2/10) + (6/100) is equal to the decimal number 9.26.
Fractions with denominators 10,100, etc. can be written in a form, using a decimal point, called decimal numbers or decimals.
9 + (2/10) + (6/100) = 9 + 0.2 + 0.06
= 9.26

Q.14 Decimal 16.25 is equal to the fraction ______.

Ans.
Decimal 16.25 is equal to the fraction 16¼ or 65/4.
Decimals can be converted into fractions by removing their decimal points and writing 10,100, etc in the denominators, depending upon the number of decimal places in the decimals.
16.25 = 1625/100
Divide both numerator and denominator by 25.
= 65/4
= 16¼

Q.15 Fraction 7/25 is equal to the decimal number ______.

Ans.
Fraction 7/25 is equal to the decimal number 0.28.
Multiply numerator and denominator by 4 to get denominator 100.
(7/25) = (7 × 4)/(25 × 4)
=28/100
We know that, fractions with denominators 10,100, etc. can be written in a form, using a decimal point, called decimal numbers or decimals.
= 0.28

Q.16 (17/9) + (41/9) = .

Ans.
(17/9) + (41/9) = 58/9.
Fractions with same denominators are called like fractions.
Sum of two like fractions = (17 + 41)/9
= 58/9

Q.17 (67/14) – (24/14) = .

Ans.
(67/14) – (24/14) = 43/14.
Fractions with same denominators are called like fractions.
Difference of two fractions = (67 – 24)/14
= 43/14

Q.18 17/2 + 3½ = .

Ans.
17/2 + 3½ = 12.
First we have to convert mixed fraction into improper fraction = 3½ = 7/2
Fractions with same denominators are called like fractions.
Sum of two like fractions = (17/2) + (7/2)
= (17 + 7)/2
= 24/2
= 12

Q.20 9 ¼ – 5/4 = _________.

Ans.
9 ¼ – 5/4 = 37/4 – 5/4 = (37 – 5)/4 = 32/4 = 8.

Q.21 4.55 + 9.73 = ______.

Ans.
4.55 + 9.73 = 14.28.

Q.22 8.76 – 2.68 = ______.

Ans.
8.76 – 2.68 = 6.08.

Q.23 The value of 50 coins of 50 paisa = ₹______.

Ans.
The value of 50 coins of 50 paisa = ₹25.
We know that, ₹ 1 = 100 paisa
So, 50 coins of 50 paisa = 50 × 50
= 2500 paisa.
Then,
= 2500/100
= ₹ 25

Q.24 3 Hundredths + 3 tenths = ______.

Ans.
3 Hundredths + 3 tenths = 0.33.
Place value of the place immediately after the decimal point (i.e. tenth place) is 1/10, that of next place (i.e. hundredths place) is 1/100 and so on.
3 Hundredths is written as = 3 × (1/100)
= 0.03
3 tenths is written as = 3 × (1/10)
= 0.3
Then sum of 3 Hundredths, 3 tenths = 0.03 + 0.3
= 0.33

Q.25 Fractions with same numerator are called like fractions.

Ans.
False. Fractions with same denominators are called like fractions.

Q.26 Fraction 18/39 is in its lowest form.

Ans.
False. Lowest form of given fraction 18/39
Divide both numerator and denominator by 3,
= 6/13

Q.27 Fractions 15/39 and 45/117 are equivalent fractions.

Ans.
True. Consider the two given fractions, 15/39 and 45/117
So, (15/39) = (45/117)
By cross multiplication, we get
(15 × 117) = (45 × 39)
1,755 = 1,755

Q.28 The sum of two fractions is always a fraction.

Ans.
True. For example: consider two fractions 10/5 and 15/5.
Sum of two fractions = (10 + 15)/5
= 25/5
= 5
= 5/1
A fraction in which there is no common factor, except 1, in its numerator and denominator is called a fraction in the simplest or lowest form.
When 2 fractions are added, the result in most cases will be a fraction p/q form, but in some case if it does happen to be just an integer, it can always be written with denominator 1 (hence p/q form).

Q.29 The result obtained by subtracting a fraction from another fraction is necessarily a fraction.

Ans.
False. Not necessarily a fraction. But can be written in fraction.

Q.30 If a whole or an object is divided into a number of equal parts, then each part represents a fraction.

Ans.
True. A fraction is a number representing a part of a whole. This whole may be a single object or a group of objects.
For example: consider a circle is divided into 4 equal parts. Out of four equal parts 3 of them are shaded.
So, it can be represented in the form of fraction = 3/4

Q.31 The place value of a digit at the tenths place is 10 times the same digit at the ones place.

Ans.
False. Let us assume a digit be ‘y’.
The place value of a digit at the tenths place = y × (1/10)
= y/10
Then,
The tenths place is 10 times the same digit at the ones place.
y/10 = 10y is not possible.

Q.32 The place value of a digit at the hundredths place is 1/10 times the same digit at the tenths place.

Ans.
True Let ‘a’ be the same digit at tens and hundreds place in a number.
Place value of digit at tens place = 10 × a = 10a
Place value of digit at hundreds place = 100 × a = 100a
Hence, the place value of a digit at the hundreds place is 10 times the same digit at the tens place.

Q.33 The decimal 3.725 is equal to 3.72 correct to two decimal places.

Ans.
False. Consider the given decimal number, 3.725
The thousandths place has number 5.
Then, hundredths has number 2 it will be increased by 1 number to correct two decimal places.
Therefore, the decimal 3.725 is equal to 3.73 correct to two decimal places.

Q.34 In the decimal form, fraction 25/8 = 3.125

Ans. True. 25/8 can be further simplified by dividing both numerator and denominator by 8. n= 3.125

Q.34 The decimal 23.2 =

Ans.
False. The decimal 23.2 = 232/10
Dividing both denominator and numerator by 2, we get.
= 116/5
=

Q.35 The fraction represented by the shaded portion in the adjoining figure is 3/8.

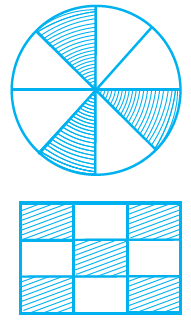
Ans.
True. Circle is divided into 8 equal parts. Out of 8 equal parts 3 of them are shaded.

Q.36 The fraction represented by the unshaded portion in the adjoining figure is 5/9.

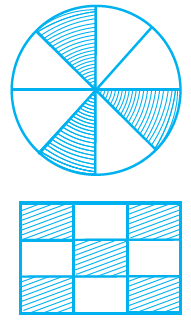
Ans.
False. Rectangle is divided into 9 equal parts. Out of 9 equal parts 4 of them are unshaded.
So, fraction represented by the unshaded portion in the adjoining figure = 4/9.

Q.37 (25/19) + (6/19) = 31/38

Ans.
False.
So, (25/19) + (6/19)
= (25 + 6)/19
= 31/19
Hence, 31/19 ≠ 31/38

Q.38 (8/18) – (8/15) = 8/3

Ans.
False. Consider Left Hand Side (LHS),
LCM of 18 and 15 = 90
Then,
(8/18) = (8 × 5)/(18 × 5) = 40/90
(8/15) = (8 × 6)/(15 × 6) = 48/90
Difference of two fractions (40/90) – (48/90)
= -8/90
Right Hand Side (RHS) = 8/3
By comparing LHS and RHS,
LHS ≠ RHS
-8/90 ≠ 8/3

Q.39 (7/12) + (11/12) = 3/2

Ans.
True. Consider Left Hand Side (LHS),
Sum of like fractions = (7/12) + (11/12)
= (7 + 11)/12
= 18/12
Divide both numerator and denominator by 6, we get,
= 3/2
Right Hand Side (RHS) = 3/2
By comparing LHS and RHS,
LHS = RHS
3/2 = 3/2

Q.40 3.03 + 0.016 =3.019

Ans.
False. First we have to convert given decimals into like decimals = 3.030 and 0.016
Sum of two decimals = 3.030 + 0.016 = 3.046

Q.41 42.28 – 3.19 = 39.09

Ans.
True. Subtracting 3.19 from 42.28,
42.28
– 3.19
39.09

Q.42 (16/25) > (13/25)

Ans.
True. Given two fractions are like fractions,
Fractions with same denominators are called like fractions.
So, 16 > 13
Therefore, (16/25) > (13/25)

Q.43 19.25 < 19.053

Ans.
False.
By comparing tenths place of both fractions = 2 > 0
Therefore, 19.25 > 19.053

Q.44 13.730 = 13.73

Ans.
True. First we have to convert given decimals into like decimals = 13.730
So, 13.730 = 13.730
Therefore, 13.730 = 13.73